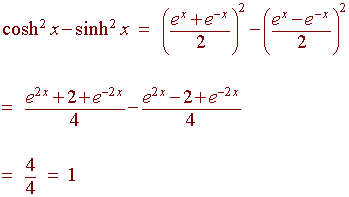
Please Subscribe here thank you. We hope it will be very helpful for you and it will help you to understand the solving process.

The quotient rule is just as easy.
Derivative of sinh 2x. Derivative of sinh 2x full pad. X msquare log_ msquare sqrt square nthroot msquare square le. Using the chain rule the derivative of sin2x is 2sinxcosx Note using the trigonometric identity 2cosxsinx sin2x the derivative of sin2x can also be written as sin2x Finally just a note on syntax and notation.
Sin2x is sometimes written in the forms below with the derivative as per the calculations above. Gx sinh2 x Find the derivative About Press Copyright Contact us Creators Advertise Developers Terms Privacy Policy Safety How YouTube works Test new features 2021 Google LLC. Find the Derivative - ddx sin h 2x sinh 2x sinh 2 x Differentiate using the chain rule which states that d dx f gx d d x f g x is f gxgx f g x g x where f x sinhx f x sinh x and gx 2x g x 2 x.
Tap for more steps. Likewise what is the derivative of Sinh 2x. The derivative of sinhu sinh u with respect to u u is coshu cosh u.
Replace all occurrences of u u with 2x 2 x. In respect to this what is Sinhx differentiation. It is known that the hyperbolic sine and cosine are connected by the relationship.
Sinhx ex ex 2. Ex 2 ex 2. Dsinhx dx d dx ex 2 ex 2 d dx ex 2 d dx ex 2 by the sum rule.
Ex 2 ex 2 by basic differentiation of exponential functions. Ex ex 2 coshx. The quotient rule is just as easy.
Let u ex ex and v 2. Derivative of sinh cosh x full pad. X msquare log_ msquare sqrt square nthroot msquare square le.
Derivatives of Hyperbolic Sine and Cosine Hyperbolic sine pronounced sinsh. Ex ex sinhx 2 Hyperbolic cosine pronounced cosh. E x e coshx 2 d x sinhx d e ex ex e x coshx dx dx 2 2 Likewise d coshx sinhx dx d Note that this is different from cosx dx.
2 x e x e x e x e x 2 2 e x e x 2 e x e x 2 2 sinh. Follow this answer to receive notifications. Answered Dec 26 19 at 1032.
Solution to Example 2. Let g x 2 sinh x and h x 4 cosh x function f is the sum of functions g and h. F x g x h x.
Use the sum rule f x g x h x to find the derivative of function f. Simple step by step solution to learn. Simple and easy to understand so dont hesitate to use it as a solution of your homework.
Below you can find the full step by step solution for you problem. We hope it will be very helpful for you and it will help you to understand the solving process. In this video I go over the derivations of the double angle or double argument identities for hyperbolic trig cosine and sine namely cosh2x and sinh2x.
Derivatives of Inverse Hyperbolic Functions. Consider now the derivatives of 6 inverse hyperbolic functions. The corresponding differentiation formulas can be derived using the inverse function theorem.
Take for example the function y fleft x right textarcsinhx inverse hyperbolic sine. Together with the function x varphi left y right sinh y they form a pair of mutually. Derivative of ln sinh x vs derivative of sinh ln x - YouTube.
Let u x 2 and y sinh u and use the chain rule to find the derivative of the given function f as follows. F x dy du du dx dy du cosh u see formula above and du dx 2 x. Given a function there are many ways to denote the derivative of with respect to.
The most common ways are and. When a derivative is taken times the notation or is used. These are called higher-order derivatives.
Note for second-order derivatives the notation. Using u sinx gives us y u2. Dy dx dy du du dx.
Dy du 2u. Du dx cosx. Dy dx 2ucosx 2sinxcosx.
Please Subscribe here thank you. HttpsgooglJQ8NysPartial Derivatives of z sinh2x 3y. Fracddxecosh 2xecosh 2xsinh 2x2 fracddxecosh 2x Applying the chain rule fracdfudxfracdfducdot fracdudx Letcosh 2xu.
Each of the functions sinh and cosh is equal to its second derivative that is. D 2 d x 2 sinh x sinh x displaystyle frac d2dx2sinh xsinh x d 2 d x 2 cosh x cosh x. Displaystyle frac d2dx2cosh xcosh x.
Proof of tanhx 1 - tanh 2 x. From the derivatives of sinhx and coshx. For the first derivations we have.
Cosh 2x 2sinh 2x using g ffg f So we suppose that the nth derivative is 2nsinh 2x if n is not an even integer else The derivative it equal to 2ncosh 2x so we shoul to treat the tow cases when n is even and when is not. In this section we define the hyperbolic functions give the relationships between them and some of the basic facts involving hyperbolic functions. We also give the derivatives of each of the six hyperbolic functions and show the derivation of the formula for hyperbolic sine.
Wanna do this in one min.